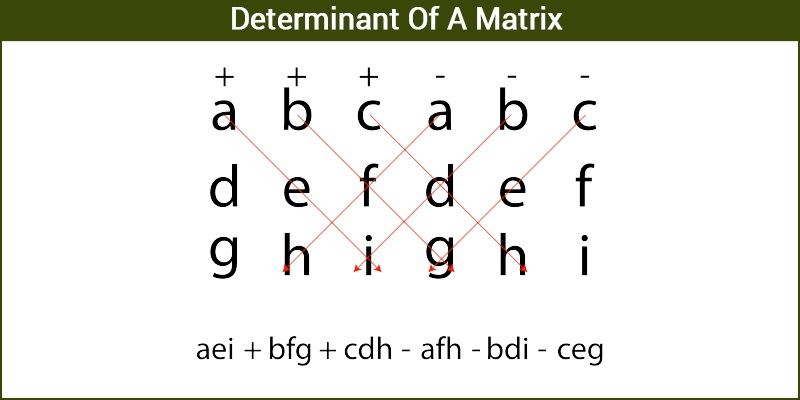
Frameworks and determinants date back to the mid second century BC, in spite of the fact that follows can be followed back to the fourth century BC. In any case, it was only after the finish of the seventeenth century that the thoughts returned and improvement was truly in progress.
It ought to shock no one that networks and determinants probably started through the investigation of frameworks of straight conditions. The Babylonians concentrated on issues that together lead to direct conditions and a portion of these are protected in earth tablets that make due. For instance a tablet from around 300 BC has the accompanying issue:-
One bushel for each square yard. Assuming the all out yield is 1100 bushels, what is the size of each ranch.
Click here https://caresclub.com/
Between 200 BC and 100 BC the Chinese drew a lot nearer to frameworks than to the Babylonians. As a matter of fact saying that the text of nine parts on numerical craftsmanship, composed during the Han line, gives the primary known illustration of lattice methods is fair. Initial an issue is set up which is like the Babylonian model above:-
There are three kinds of maize, of which three heaps of the initial, two of the second and one of the third have 39 measures. Two of the initial, three of the second and one of the third measure 34. Also, complete 26 proportions of perhaps the earliest, two of the second and three of the third. What number of corn sizes are in a heap of each sort?
know more about these kinds of stuff here 75 inches in cm
Our late twentieth century techniques would have permitted us to compose straight conditions as lines of frameworks rather than segments, obviously the strategy is something similar. Most strikingly the writer, writing in 200 BC, trains the peruser to duplicate the center segment by 3 and to take away the right section whatever number times as could be expected under the circumstances, then, at that point, to deduct the right section from the initial 3 fold the number of times as could be expected. Is. segment
Cardon, in Ars Magna (1545), gives a standard for settling an arrangement of two straight conditions which he calls regula di modo and [7] the mother of the principles! This standard makes sense of what is basically Cramer’s regulation for tackling a 2 × 2 framework, albeit the cardan doesn’t shape the last step. So Kardon doesn’t arrive at the meaning of a determinant, yet, from the advantage of vision, we can see that his strategy prompts the definition.
A significant number of the standard consequences of early lattice hypothesis showed up well before grids were the object of numerical examination interestingly. For instance, de Mind in the Components of Bends, distributed as a feature of the Discourses on the 1660 Latin release of Descartes’ Math, demonstrated the way that the change of tomahawks can lessen a given condition to a sanctioned structure for a cone. gives. This is identical to diagonalizing a symmetric lattice yet de Mind never considered it in these terms.
The possibility of the determinant showed up in Japan before it showed up in Europe. In 1683 Seki composed a technique for taking care of dispersing issues in which grid strategies were composed as tables, similarly as the Chinese strategies depicted previously. Without a term that compares to ‘determinant’, Seki actually presented determiners and gave general techniques to compute them in light of models. Utilizing his ‘determinants’ Seki had the option to track down the determinants of 2 × 2, 3 × 3, 4 × 4 and 5 × 5 lattices and applied them to settle conditions yet not frameworks of direct conditions.
Leibniz was persuaded that great numerical documentation was the way to advance so he explored different avenues regarding various documentations for coefficient frameworks. There are in excess of 50 unique approaches to composing the coefficient framework in his unpublished compositions, which he dealt with during a time of 50 years starting in 1678. Just two distributions (1700 and 1710) contain results on coefficient frameworks and utilize a similar documentation as their paper. Referenced above from De l’Hopital.
Leibniz has utilized the term ‘considerable’ for specific mixes of terms of the determinant. He demonstrated different outcomes on results, including basically Cramer’s regulation. He likewise realize that a determinant could be extended utilizing any section — presently called the Laplace development. As well as concentrating on coefficient frameworks of conditions, Leibniz likewise concentrated on coefficient frameworks of quadratic structures which normally prompted grid hypothesis.
Maclaurin composed the Composition of Polynomial math during the 1730s, in spite of the fact that it was not distributed until 1748, two years after his demise. It remembers recently distributed results for determinants demonstrating Cramer’s regulation for 2 × 2 and 3 × 3 frameworks and showing how the 4 × 4 case would work. Cramer gave the basic principle for n \times nn×n frameworks in a paper Prologue to the Examination of Logarithmic Bends (1750). It emerged from the craving to find the condition of a plane bend going through various given focuses. The standard shows up in the supplement to the paper yet no verification is given:-
The worth of every obscure is found by making n parts of which the shared factor has however many terms as there are changes of n things.
CReimer proceeds to make sense of how one works out these terms as results of specific coefficients in conditions and how one decides the sign. He additionally expresses how to get n parts of portions by subbing a portion of the coefficients in this computation by the framework’s consistent terms.
Capabilities on determiners presently started to consistently show up. In 1764 Bezout gave techniques for working out determinants, as did Vandermonde in 1771. In 1772 Laplace guaranteed that the techniques presented by Cramer and Bezout were unreasonable and, in a paper where they concentrated on the circles of the inward planets, they examined the arrangement of the framework directly without really registering it utilizing determinants. the condition. Rather shockingly Laplace utilized the term ‘considerable’ which we presently call deterministic: shockingly since it is a similar term utilized by Leibniz, Laplace probably been uninformed about Leibniz’s work. . Laplace gave the expansion of a determinant which is currently named after him.
Lagrange read up characters for 3 × 3 utilitarian determinants in a 1773 paper. Anyway this remark has been made with folly in light of the fact that Lagrange himself saw no association between his work and that of Laplace and Vandermonde. In this 1773 paper on mechanics, notwithstanding, we presently consider a determinant an amount understanding interestingly. Lagrange showed that the tetrahedron is framed by O(0,0,0) and three focuses M(x,y,z), M'(x’,y’,z’), M”(x”,y) Is. ”, z”) m(x, y, z), m
The term ‘determinant’ was first presented by Gauss in Quite a while Math (1801) alluding to quadratic structures. He utilized the term on the grounds that the determinant decides the properties of the quadratic structure. Anyway the idea isn’t equivalent to our determinant. In a similar work Gauss gives the coefficients of their quadratic structures in rectangular clusters. He depicts grid duplication (which he considers sythesis so he has not yet arrived at the idea of network variable based math) and inverses of a framework with extraordinary reference to varieties of coefficients of quadratic structures.
The Gaussian end, which previously showed up in a nine-part text on numerical craftsmanship written in 200 BC, was utilized by Gauss in his work concentrating on the circle of the space rock Pallas. Utilizing Pallas’ perceptions taken somewhere in the range of 1803 and 1809, Gauss determined an arrangement of six straight conditions in six questions. Gauss gave a precise strategy for settling such conditions which is Gaussian end on the coefficient framework.
It was Cauchy who involved ‘determiner’ in its advanced sense in 1812. Cauchy’s work is the most over the top total of the early deals with determinants. He exposed before results and forced new aftereffects of his own on minors and everyone around him. In a 1812 paper the duplication hypothesis for determinants is demonstrated interestingly, in any case, at a similar gathering of the Institut de France, Binet likewise read a paper which contained a proof of the duplication hypothesis yet was less good than that given by Cauchy. ,
In 1826, Cauchy utilized the term ‘table’ for a framework of coefficients with regards to quadratic structures in n factors. He tracked down the eigenvalues and gave results on the diagonalization of a lattice as far as changing a structure into an amount of squares. Cauchy additionally presented the possibility of comparative networks (however not terms) and showed that in the event that two frameworks are equivalent, they have a similar trademark condition. He again demonstrated as far as quadratic structures that each genuine symmetric lattice is diagonalizable.
Jacques Sturm gave a speculation of the eigenvalue issue concerning tackling frameworks of normal differential conditions. As a matter of fact the idea of an eigenvalue seemed quite a while back, again by D’Alembert concentrating on the movement of a string with mass joined to it at different places, dealing with frameworks of straight differential conditions.
It ought to be underlined that neither Cauchy nor Jacques Sturm understood the monstrosity of the thoughts they were offering and saw them just in the particular settings in which they were working. Jacobi around 1830 and again in 1850 and 1860 Kronecker and Weierstrass likewise saw network results, yet again in an extraordinary setting, this time the thought of a direct change. Jacobi distributed three compositions on determinants in 1841. These were significant in that interestingly the meaning of the determinant was made in an algorithmic manner and the sections in the determinant were not determined, so their outcomes were similarly well relevant to situations where the passages were numbers or where they were capabilities. . These three papers by Jacobi spread the word.