In math, a decagon is known as a ten-sided polygon or ten-gon. The amount of the inside points of a normal hexagon is 1440° and the amount of the outside points of a decimal is 360°.
Tipsfeed tipsfeed.com
Significance Of Offering
A decagon is a ten-sided polygon with ten vertices and ten points. In this way, an offering shape can be characterized as a polygon with ten sides, ten inside points, and ten vertices. In view of the sides of an offering, they are comprehensively characterized into standard decagons and unpredictable decagons. A standard offering has 35 diagonals and 8 triangles. The places of these diagonals and triangles are made sense of in later segments of this article.
15 of 1500 https://tipsfeed.com/15-of-1500/
Kind Of Decimal
Decimals can be delegated ordinary and unpredictable decimals, contingent upon the side-length and point estimations. There are three potential groupings of decimals which are given underneath:
normal and unpredictable decimals
Curved and Inward Decimal
straightforward and complex decimals
normal offering
A normal decagon is a polygon with 10 equivalent sides and 10 vertices. Sides and points are harmonious in a standard hexagon. The qualities of a normal offering are:
Every one of the sides are of equivalent length and in a normal decagon all points are equivalent in measure.
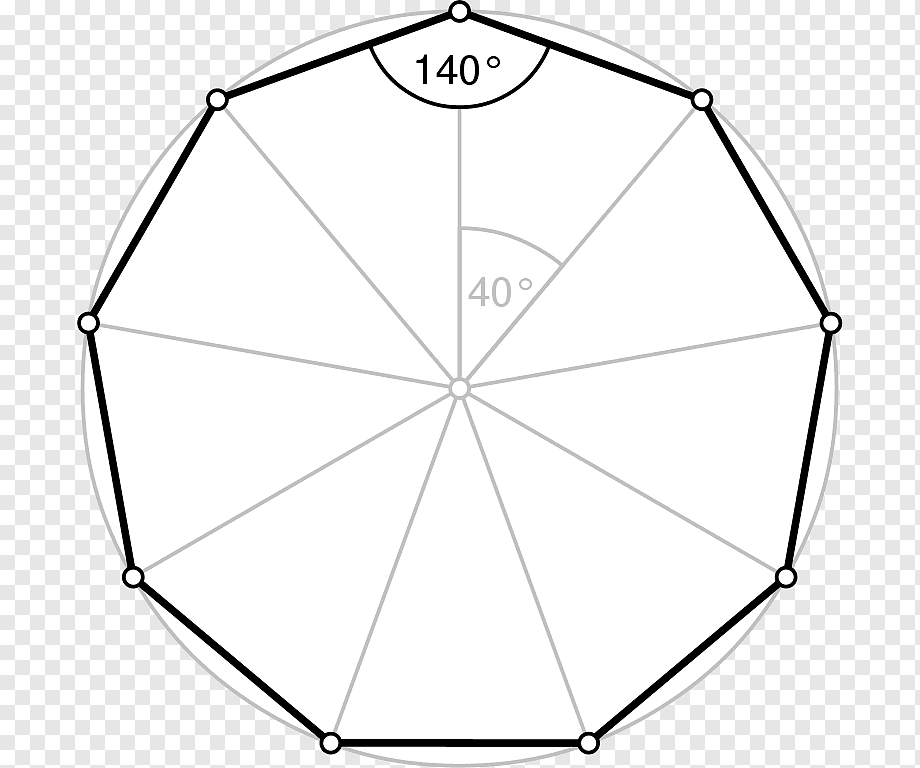
The proportion of every inside point in ordinary decimal is 144º, while the proportion of every outside point is 36º.
ordinary offering
unpredictable decadal
An unpredictable decimal doesn’t have equivalent sides and points. Estimations have somewhere around two unique sides and points. Take a gander at the photos underneath which show unpredictable decimals.
unpredictable decadal
Curved and Inward Decimals
Like some other polygon, decimals can be either curved or inward. A raised decimeter juts outward in light of the fact that all inside points are under 180°. Though sunken decagons have space (a profound break). In curved a very long time no less than one inside point is more prominent than 180°.
curved and inward offering
straightforward and complex decimals
Straightforward decagons allude to many years whose no arm cuts itself. They follow every one of the above normal decimal principles. Though mind boggling decagons allude to decimals that are self-converging and have extra interior spaces. They rigorously observe no set guidelines of decimals in regards to their inside points and their aggregate.
Straightforward And Complex Decimals
Properties Of Offering
A portion of the significant properties of the many years are recorded here.
The amount of the inside points is 1440°.
The amount of the proportions of an outside point is 360°.
On account of a normal offering, the focal point estimates 36 degrees.
There are 35 diagonals in a 10th.
There are 8 triangles in a 10th.
the amount of the inside points of the ten years
To track down the amount of the inside points of a quadrilateral, initial gap it into triangles. There are eight triangles in an ordinary 10th. We realize that the amount of the points of each and every triangle is 180°. So 180° × 8 = 1440°. In this way, the amount of the relative multitude of inside points of a decimal is 1440°.
We realize that the quantity of sides of a decimal is 10. Thusly, we partition the amount of the inside points by 10.
1440° 10 = 144°
Subsequently, one of the inside points of a normal offering shape is 144°. Furthermore, the amount of the multitude of inside points of a 10th is 1440°.
Estimation Of Focal Points Of A Standard Decimal
To find the proportion of the focal point of a normal decimeter, we really want to attract a circle the center. A circle makes 360°. Partition it by ten, since there are 10 sides in a 10th. 360° 10 = 36°. In this way, the proportion of the focal point of a standard hexagon is 36°.
mid point
Tithe Corner To Corner
A corner to corner is a line that can be attracted starting with one vertex then onto the next. The quantity of diagonals of a polygon is determined by: n(n−3) 2. In the decagon, n is the quantity of sides that is equivalent to 10, so n=10. presently we get,
n(n−3) 2 = 10(10−3) 2
Consequently, the quantity of diagonals in a decagon is 35.
There Are 8 Triangles In The 10th
Assuming one vertex is gotten together with the leftover vertices of the hexagon, 8 triangles will be framed. Assuming all the vertices are joined autonomously, 80 triangles (8×10) will be framed. Take a gander at the figure beneath, which shows the diagonals and triangles of a decimal.